
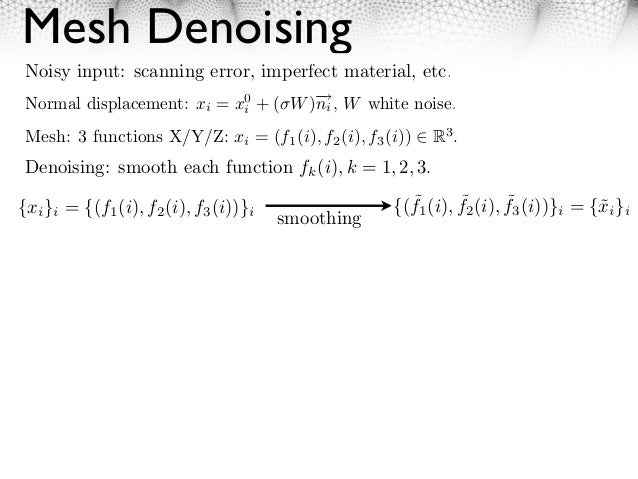
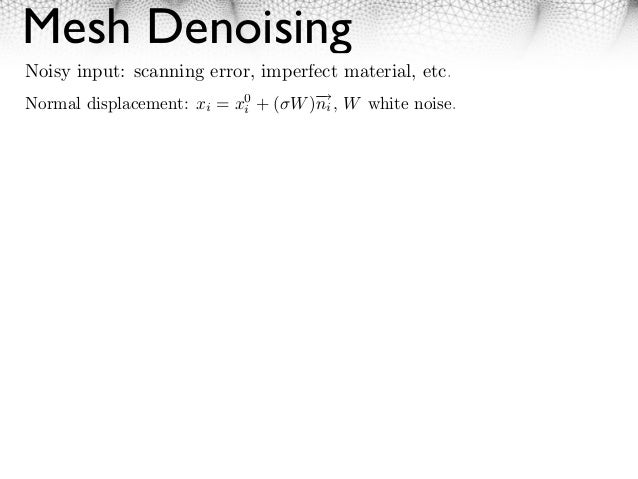
In explicit numerical methods, a severe limitation on the time step is often due to stability restrictions imposed by the CFL condition. This work concerns the development of a meshfree semi-implicit numerical scheme based on the Smoothed Particle Hydrodynamics (SPH) method, here applied to free surface hydrodynamic problems governed by the shallow water equations. With these new visualization approaches, new insights for the quantitative comparison of fragmentation and wave propagation become intuitively accessible. For a quantitative study with a simple geometry, the edge-on impact experiment (Schradin, Scripts German Acad Aeronaut Res 40:21–68, 1939 Strassburger, Int J Appl Ceram Technol 1:1:235–242, 2004 Kawai et al., Procedia Eng 103:287–293, 2015) can be applied. For the extraction and visual analysis, we used the widely-used Stanford bunny as a complex geometry. Here, the particle-based data is re-sampled and rendered with standard volume rendering techniques to address the interference pattern of the stress wave after reflection at the boundary. The second approach focuses on the visualization of the stress after an impact. Army Research Laboratory, 2005), Vogler et al. The distribution of the fragment size can be obtained by real-world experiments as demonstrated in Schram and Meyer (Simulating the formation and evolution of behind armor debris fields. The first analysis technique is an extended component labeling algorithm to extract the fragment size and the corresponding histograms. As the underlying simulation model we consider the particle method Peridynamics, a non-local generalization of continuum mechanics. We present two visualization techniques to post-process particle-based simulation data, and we highlight new aspects for the quantitative comparison with experimental data. The analysis of simulation results and the verification against experimental data is essential to develop and interpret simulation models for impact damage.
However, the implementation of meshfree methods and their parallelization also requires special attention, for instance with respect to numerical integration. Furthermore, meshfree methods have a number of advantageous features that are especially attractive when dealing with multiscale phenomena: A-priori knowledge about the solution’s particular local behavior can easily be introduced into the meshfree approximation space, and coarse scale approximations can be seamlessly refined by adding fine scale information.

For instance, meshfree methods can be viewed as a natural extension of classical finite element and finite difference methods to scattered node configurations with no fixed connectivity. The growing interest in these methods is in part due to the fact that they offer extremely flexible numerical tools and can be interpreted in a number of ways. There have been substantial developments in meshfree methods, particle methods, and generalized finite element methods since the mid 1990s.


 0 kommentar(er)
0 kommentar(er)
